The Speed of Light in the Great Pyramid

The following post is the beginning of a paper of mine that was accepted for publication in the scientific journal Archaeological Discovery. If it catches your fancy, there is a link to the full paper at the end of this excerpt...

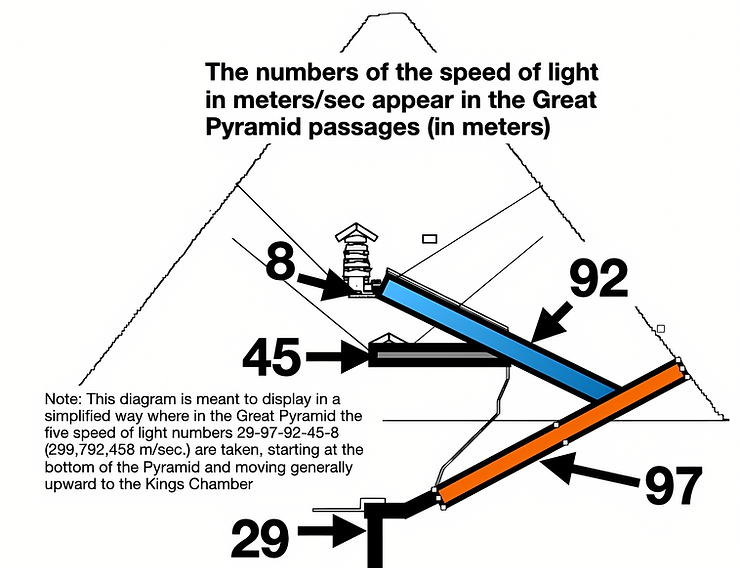
Figure 1

Abstract

Measurements in meters taken from known and prominent features of the Great Pyramid of Giza, when placed in a rational order based on the orientation and common understanding of its existing passage systems, yield the numbers in the speed of light given in meters per second in a vacuum: 299,792,458. The meter measurements found in this study each contain two digits, except for the final one, which is a single digit, as follows: 29 – 97 – 92 - 45 and 8. This paper examines the basis in the Great Pyramid for each of these numbers. A discussion of various issues related to this speed of light finding follows.

Introduction

It is certainly an awkward and unusual claim that the speed of light numbers can be derived by attention to the measures of the passages of the Great Pyramid. I can find nowhere in the body of literature on the Great Pyramid, including the writing of those known pejoratively by many Egyptologists as “pyramidologists”, where this claim is advanced. But this is indeed the scientific claim being advanced here. This thesis is aided first, by the fact that the five speed of light numbers, 29, 97, 92, 45 and 8, are derived from all the traditional and well known passages and chambers of the Pyramid. None are left out. And secondly these five numbers follow the rational sequence of moving from the lowest part of the Pyramid to the highest.

After the architectural and metrological arguments for the existence of the speed of light numbers, several issues are discussed. What about the claims of new passages being found in the Pyramid that are thus not addressed by these numbers? How can it be explained that these numbers appear through use of the metric system, supposedly a relatively recently created measuring system? How much is the thesis degraded by the fact that all the speed of light numbers come through rounding the final measures in each Pyramid section? Did the ancient Egyptians have advanced scientific knowledge?

The discussion includes new understandings gained by the author in pursuit of this inquiry, including the findings related to the upper and lower mouths of the Well Shaft, and the division of all the various Pyramid passages into two “roads”, as referenced in the Book of the Dead.

Genesis

While others have posited the finding of the modern speed of light constant in various ways in the Great Pyramid (see Appendix), this is not what prompted the current inquiry. Science smiles at, but allows, the story of Isaac Newton sitting under an apple tree in his garden at Woolsthorpe Manor and somehow being inspired to theorize about the nature of gravity from the event of an apple falling from that tree. The current inquiry was begun when an engineer, Bob Crielly, an associate of the American Institute for Pyramid Research, had a dream of the Great Pyramid passage lengths producing the speed of light. This was the apple falling from the tree for me. I went to the best measures of the Great Pyramid passages I could find and began to see if the numbers in the speed of light were somehow tucked away in the Great Pyramid passages.

Mr. Crielly, as an engineer, usually uses the metric system when corresponding with me about various pursuits in which we are engaged. While it may immediately disqualify the present inquiry in the minds of some that an ancient structure might encode a measure founded in 1799 by the French, I nonetheless chose to look for speed of light numbers in meters. The world’s leading metrologists have reviewed the possibility that the meter has a history preceding the enlightened French creation of the metric system but have reached no consensual conclusion. But some of the metrologists allow a history of the meter that starts before 1799.  Let me give one example of this finding, not in the way of proof, but to show a rational basis for the assertion.

Richard Heath in Sacred Number and the Origins of Civilization writes:

“Who would have suspected that the new measure of the meter, designed to replace all the measures of the ancient world, had been based upon an actual ancient measure used in France during megalithic times?” (R. Heath 2006)

John Neal in his metrological study “All Done with Mirrors” says that it was an epochal discovery of the metrologists when it was realized that most ancient systems of measure were connected by integral ratios. He writes:

“It was at this point that it was realized that Sumerian, Egyptian, Greek and English metrology were, in fact, one system, and a comparative yardstick was at hand to categorize all other systems and values.” (Neal 2000)

Progression of Various Feet Measures Leading to the Meter
	Foot Length	Ratio Connecting to the next measure
Iberian Foot	32/35 feet	Connection to the Roman foot: 21/20
Roman Foot	24/25 feet	Connection to the English foot: 25/24
English Foot	1 foot	Connection to the Persian foot: 21/20
Persian Foot	21/20 feet	Connection to the Carnac foot: 25/24
Carnac Foot	35/32 feet	Connection to the meter: 1/3
The Meter	3.281 feet (rounded)	3 times the Carnac foot = 3.281’

 Table 1. gives one example of the integral ratio connection between major systems of measure, which includes in its final iteration a connection to the meter.

Another justification for using the meter to measure the Great Pyramid passages is its apparent use in two places in the Kings Chamber. The first is in the perimeter of that sacred space. It is well known that the Chamber is a double square with dimensions of 10 royal cubits by 20 royal cubits. The perimeter in cubits is thus an even 60 cubits. Converting that distance to meters yields 31.416 meters, which as a number is π (pi) times 10. Since it is widely recognized that the Great Pyramid is built on π proportions, for instance, its height being in 4/π proportion to a half base of unity (and a slope angle in φ proportion), then a Kings Chamber perimeter of 10π meters could be seen as intentional. The intentionality can’t be proven, but this dimension provides a rational basis for believing it could be so.

Figure 2

A second apparent use of the meter in the Kings Chamber is in the diagonal measure of the long north and south walls of the Chamber. The floor plan has such regular dimensions – 10 cubits by 20 cubits – one might think that the Egyptian designers would follow suit and have a ceiling height of an even 10 cubits.  But the ceiling is a very uneven 11.18 cubits. Why? Why wasn’t an even number of cubits used in the design of the Kings Chamber ceiling height? Again, intentionality can’t be proven, but it is an interesting fact that the very uneven ceiling measure of 11.18 cubits yields a diagonal on the Chamber’s long walls of almost exactly 12 meters.  Any other height would not achieve this resulting diagonal length. Reaching the conclusion that the 12 meter length of the design is intentional is entirely rational.

Figure 3

With these two examples of the appearance of the meter in the Kings Chamber, let us proceed to look at measurements in meters in the Great Pyramid passages.

Below is a diagram of the Great Pyramid showing its passages and the convention of their naming.

Figure 4

The Measures

The lengths of the passages which yield the speed of light in meters per second in a vacuum, (299,792,458 km/sec), are the following meter lengths: 29-97-92-45-8. These measures utilize all the major passages in the Great Pyramid and follow a sensible order, basically working from the bottom of the Pyramid upward.

29. The first measure starts from the current “bottom” of the Great Pyramid, the bottom of the pit (hereafter referred to as the “Pit”) that is on the middle of the east side of the Subterranean Chamber. The bottom of what was once called “the bottomless pit” cannot be perfectly ascertained. Perring gave the order to extend what was the bottom in his day – a depth he does not give us – by 50 additional feet. (Perring and Vyse 2015)   Because of the “want of a free circulation of air” the excavation, which was exceedingly difficult, was stopped at “thirty-eight feet.” (Perring and Vyse 2015) It is known that at various times in the past there was excessive debris in the Subterranean Chamber, owing to its acting as the dump yard for excavations performed higher in the Pyramid. The debris created by Perring’s dig into the Pit bottom added to this debris.

While there are records in ancient times of visits to the Subterranean Chamber, Davison, in 1763 wrote that the Descending Passage was so clogged that he could not enter.  (Edgar and Edgar 1923) In 1817 Caviglia cleared the debris so that the Subterranean Chamber could again be accessed, but with difficulty, as 20 years later Vyse reported that the Descending Passage was “much encumbered with stones and rubbish.”  (Perring and Vyse 2015)  

More debris must have entered the Descending passage in the next 28 years, perhaps from what Vyse and Perring left, because Scotland’s Royal Astronomer C. Piazzi Smyth reported in 1865 that the Descending Passage below the First Ascending Passage was so blocked with large stones that he was not able to visit the lower passages. (Edgar and Edgar 1923)  In 1881 Professor Flinders Petrie had enough of the obstructing stones moved that he was able to make the descent to the lowest Pyramid chamber and take limited measurements. The measurements he took are useful in this quest for the speed of light numbers, but they do not give the place we want to start: the bottom of the Pit. Petrie gives no measure for this.

Figure 5 summarizes Petrie’s measures. Petrie’s measures for the Descending Passage are in inches from the original entrance of the Pyramid.  To the northern lip of the Well Shaft opening into the Descending Passage, which Petrie calls the “lower mouth,” is 3825 inches. From the entrance to the beginning of the horizontal passage to the Subterranean Chamber is 4143”, thus yielding 318” (8.08 meters) from the northern mouth of the Well Shaft to the beginning of the horizontal floor. (Petrie 1990)

From that point, moving south along Subterranean Chamber horizontal passage floor to the entrance into the Subterranean Chamber, it is 346” (8.79 meters).

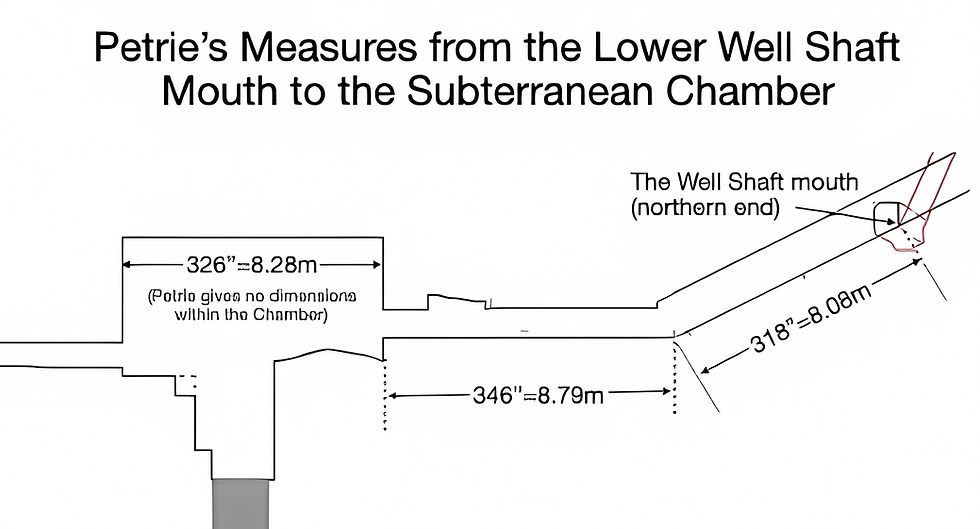
Figure 5

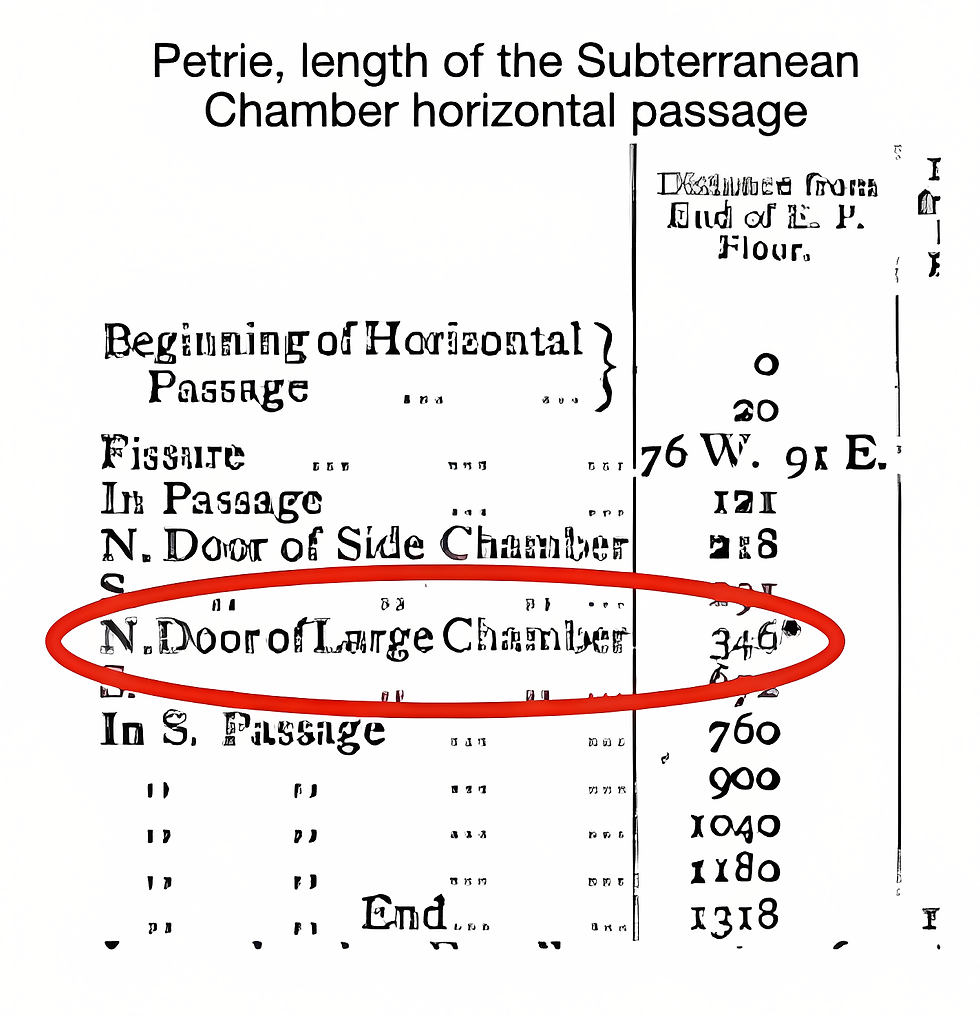
Figure 6

We are now at a point where we need measurements of the north-south width of the Pit. For this it is hard to find measures. Petrie, Rutherford, the Edgar brothers, and C. Piazzi Smyth, all known as dedicated measurers of the Great Pyramid, do not help much here, other than to let us know, as the Edgars do:

“Only approximate measures can be secured, for there is no part of the Subterranean Chamber clearly defined.” (Edgar and Edgar 1923)

Stefan Holmgren  maintains the website, “The Khufu Pyramid” (S. Holmgren n.d.) which keeps a compendium of measures and scale model drawings of the Great Pyramid from respected researchers.  Using pixels in the Pyramid drawings rendered digitally, and with Excel sheets which can compute angle and distance measures, Holmgren is able to calculate distances for parts of the Pyramid where few or no measurements exist. Here are the distances he shows for the interior sections of the Subterranean Chamber Pit which will be useful in this study (also see Figure 8)     

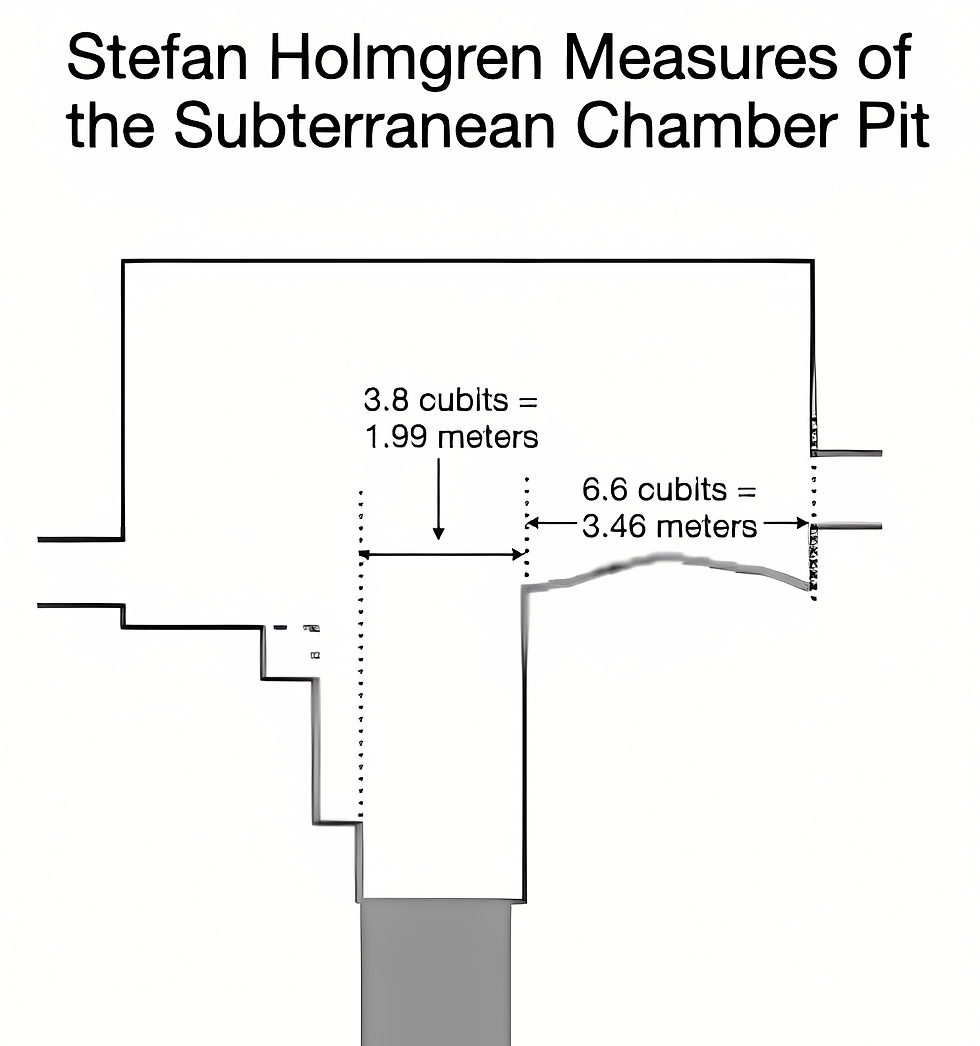
Figure 7

From the door of the Subterranean Chamber to the north end of the Pit (extended) is 3.46 meters and to the south end of the pit (extended) is another 1.99 meters for a total of 5.45 meters. Joining this with Petrie’s measures beginning with the lower mouth of the Well Shaft (Figure 5) there are 22.32 meters to the south end of the pit (extended to the floor level.)

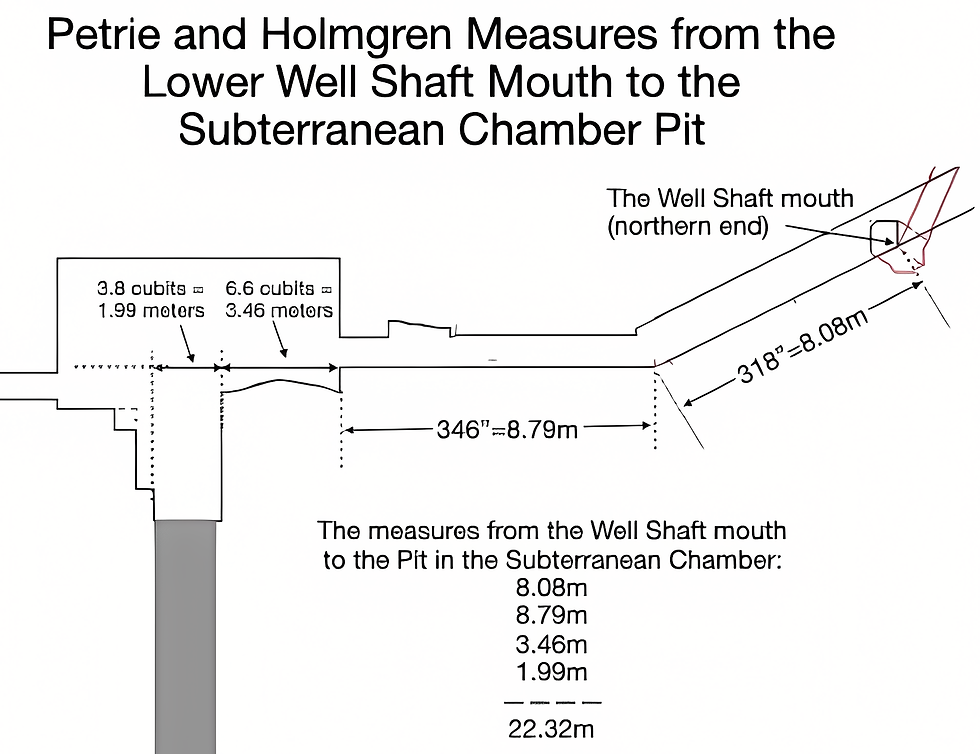
Figure 8

Since our goal was to begin the speed of light measures from the lowest portion of the Great Pyramid, the Pit within the Subterranean Chamber, we now need a measurement from the Chamber floor line down to the bottom of the Pit. The original floor there does not now exist because Perring and Vyse interpreted Herodotus to mean that the way to Pharaoh Khufu’s burial might be below this spot. As mentioned, with great effort they excavated 38 feet to no avail. The Edgar brothers, in their efforts to clear the Descending Passage and Subterranean Chamber of its debris, besides paying their workers to carry out this rubble by bags, also used this excavated hole as a dump for debris. (Edgar and Edgar 1923) Whether the current depth of this area is determined by their fill efforts, or that of others, it was this current bottom to which I pointed my laser measure when I measured it. My laser measuring tool showed a distance of 23 feet, almost exactly 7 meters (7.01), a measure I took on October 16, 2021.

Here is my photograph of the Pit, used by permission in Keith Hamilton’s study, A Layman’s Guide to the Great Pyramid, part 1. (Hamilton 2022)

Figure 9

If you want to keep reading this paper, go to the online publication here...